Lê Thị Thùy Hỏi đáp Toán 9 Toán 9 Bài tập Toán

GIUP EM VOI A

Cho biểu thức $A = \dfrac{{7\sqrt x - 2}}{{2\sqrt x + 1}}$ và $B = \dfrac{{\sqrt x + 3}}{{\sqrt x - 3}} + \dfrac{{\sqrt x - 3}}{{\sqrt x + 3}} + \dfrac{{36}}{{9 - x}}$ ( với $x \ge 0;x \ne 9$ )

a) Tính giá trị của biểu thức A khi x = 36

b) Chứng minh rằng B = 2

c) Đặt M = A - B. Tìm các giá trị nguyên của x để $\left| M \right| > M$

3

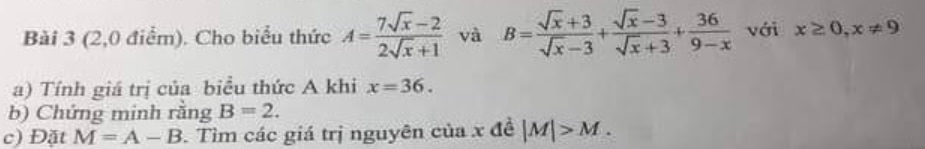
Xóa Đăng nhập để viết

3 Câu trả lời

Duy Phu
Câu2: cho biểu thức P=√4(x-1)
0 Trả lời 18/11/22
Lộc Nguyễn
√10-1
0 Trả lời 10/06/23
Biết Tuốt
a)
Khi x = 36, thay vào A ta được:
$A = \dfrac{{7\sqrt x - 2}}{{2\sqrt x + 1}} = \dfrac{{7\sqrt {36} - 2}}{{2\sqrt {36} + 1}} = \dfrac{{7.6 - 2}}{{2.6 + 1}} = \dfrac{{40}}{{13}}$
b)
$\begin{array}{l} B = \dfrac{{\sqrt x + 3}}{{\sqrt x - 3}} + \dfrac{{\sqrt x - 3}}{{\sqrt x + 3}} + \dfrac{{36}}{{9 - x}}\\ B = \dfrac{{\sqrt x + 3}}{{\sqrt x - 3}} + \dfrac{{\sqrt x - 3}}{{\sqrt x + 3}} - \dfrac{{36}}{{x - 9}}\\ B = \dfrac{{\left( {\sqrt x + 3} \right)\left( {\sqrt x + 3} \right)}}{{\left( {\sqrt x - 3} \right)\left( {\sqrt x + 3} \right)}} + \dfrac{{\left( {\sqrt x - 3} \right)\left( {\sqrt x - 3} \right)}}{{\left( {\sqrt x - 3} \right)\left( {\sqrt x + 3} \right)}} - \dfrac{{36}}{{x - 9}}\\ B = \dfrac{{x + 6\sqrt x + 9 + x - 6\sqrt x + 9 - 36}}{{\left( {\sqrt x - 3} \right)\left( {\sqrt x + 3} \right)}}\\ B = \dfrac{{2x - 18}}{{\left( {\sqrt x - 3} \right)\left( {\sqrt x + 3} \right)}}\\ \,B = \dfrac{{2\left( {x - 9} \right)}}{{\left( {\sqrt x - 3} \right)\left( {\sqrt x + 3} \right)}}\\ \,B = \dfrac{{2\left( {\sqrt x - 3} \right)\left( {\sqrt x + 3} \right)}}{{\left( {\sqrt x - 3} \right)\left( {\sqrt x + 3} \right)}}\\ B = 2 \end{array}$
c)
Ta có:
$\begin{array}{l} M = A - B = \dfrac{{7\sqrt x - 2}}{{2\sqrt x + 1}} - 2\\ M = \dfrac{{7\sqrt x - 2}}{{2\sqrt x + 1}} - \dfrac{{2\left( {2\sqrt x + 1} \right)}}{{2\sqrt x + 1}}\\ M = \dfrac{{7\sqrt x - 2 - 2\left( {2\sqrt x + 1} \right)}}{{2\sqrt x + 1}}\\ M = \dfrac{{7\sqrt x - 2 - 4\sqrt x - 2}}{{2\sqrt x + 1}}\\ M = \dfrac{{3\sqrt x - 4}}{{2\sqrt x + 1}} \end{array}$
Để $\left| M \right| > M$ thì M < 0
Mà $2\sqrt x + 1 > 0$
Nên $3\sqrt x - 4 < 0 \Leftrightarrow \sqrt x < \dfrac{4}{3} \Leftrightarrow x < \dfrac{{16}}{9}$
Kết hợp với điều kiện $x \ge 0;x \ne 9$ ta được $0 \le x < \dfrac{{16}}{9}$
0 Trả lời 14/11/22

Tìm thêm: Toán 9 Bài tập Toán

Câu hỏi mới

Danh mục

Hỏi bài ngay thôi!

Hỏi đáp Toán 9