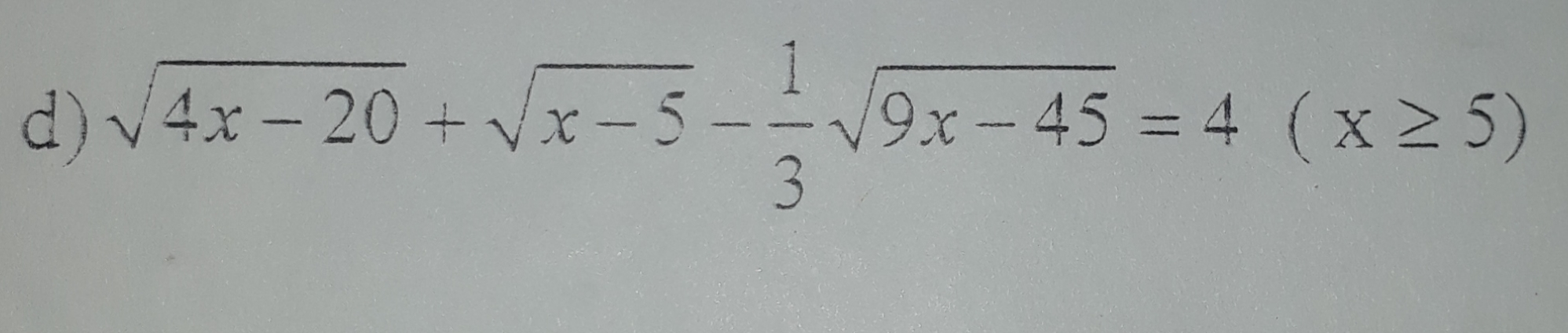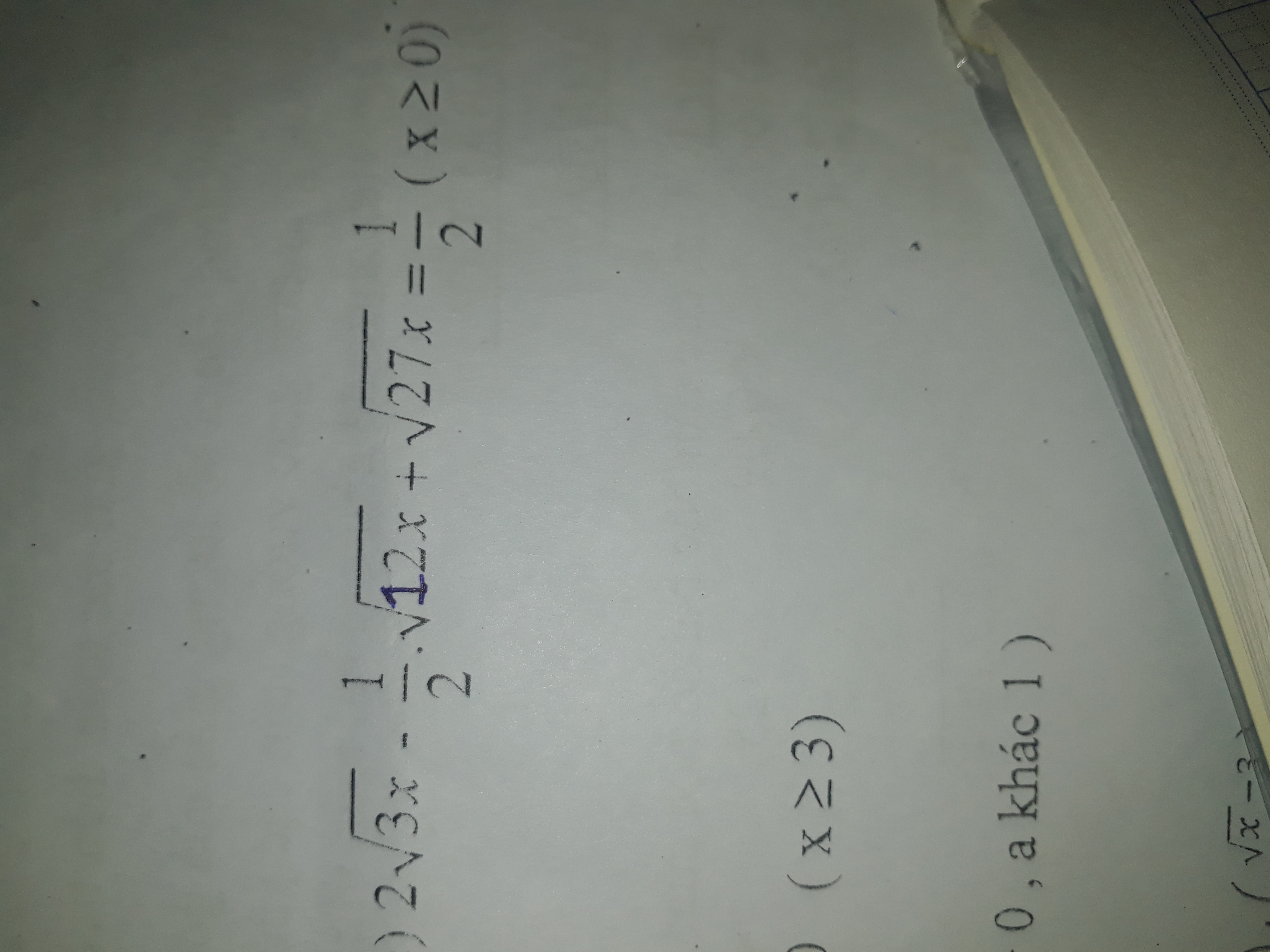Xóa Đăng nhập để viết
1 Câu trả lời
-
 Biết Tuốt0 Trả lời · 04/11/22
Biết Tuốt0 Trả lời · 04/11/22

Hỏi bài
Tìm số hạng không chứa x trong khai triển:
Ngày hỏi: Hôm qua 1 câu trả lời
luyện tập 2 trang 38 kết nối tri thức
Ngày hỏi: 3 ngày trướcGiải bài toán này
Ngày hỏi: 6 ngày trướcgiai bai
Ngày hỏi: 21:24 09/10