Giaitoan.com Toán 9 Đề thi giữa kì 1 Toán 9

Đề thi giữa kì 1 môn Toán lớp 9 - Đề số 3 Đề kiểm tra giữa kì 1 Toán 9

Nội dung
  • 1 Đánh giá

Đề thi giữa kì 1 Toán 9 - Đề số 3

Đề thi giữa kì 1 Toán 9 - Đề số 3 được Giaitoan.com biên soạn bao gồm các dạng bài tập và đáp án chi tiết được xây dựng theo trọng tâm chương trình học THCS giúp học sinh ôn tập, củng cố kiến thức, giúp định vị khả năng tư duy logic, khả năng nhận biết. Đây là nền tảng vững chắc giúp các bạn tự tin làm bài trong các kì thi và kiểm tra định kì môn Toán 9. Mời các bạn cùng tham khảo chi tiết!

A. Đề thi Toán giữa kì 1 lớp 9

Câu 1: Thực hiện phép tính

\begin{array}{l}a)\,2\sqrt 5 - \sqrt {125} - \sqrt {80} + \sqrt {605} \\b)\,2\sqrt {27} - 6\sqrt {\dfrac{4}{3}} + \dfrac{3}{5}\sqrt {75} \\c)\,\,\dfrac{2}{{\sqrt 6 - 2}} + \dfrac{2}{{\sqrt 6 + 2}} + \dfrac{5}{{\sqrt 6 }}\end{array}

Câu 2: Giải phương trình:

\begin{array}{l}a)\,2\sqrt {2x + 1} - \dfrac{3}{4}\sqrt {8x + 4} = \sqrt {\dfrac{{18x + 9}}{4}} - 3\\b)\,\,x - \sqrt {2x + 3} = 0\\c)\,\sqrt {{x^2} + 4} = \sqrt {3x + 8} \end{array}

Câu 3: Cho hai biểu thức A = \dfrac{{\sqrt x + 7}}{{\sqrt x - 1}} và B = \dfrac{1}{{\sqrt x + 2}} + \dfrac{3}{{1 - \sqrt x }} + \dfrac{{x + 8}}{{x + \sqrt x - 2}} ( với  )

a) Tính giá trị của A biết x = 16

b) Rút gọn B

c) Tìm các giá trị nguyên của x để P = A. B có giá trị nguyên

Câu 4: Một chiếc máy bay cất cánh. Đường bay lên tạo với phương ngang một góc {30^ \circ }sau khi bay được quãng đường 9 km thì máy bay đã bay lên được độ cao là bao nhiêu km theo phương thẳng đứng

Câu 5: Cho tam giác ABC nhọn có đường cao AH. Gọi M là hình chiếu của H lên AB

a) Biết AM = 3 cm; BM = 9 cm. Tính AH; HM

b) Kẻ IM vuông góc với AC tại N. Chứng minh AB. AM = AC. AN

c) Đường thẳng qua A vuông góc với MN cắt BC tại D; MN cắt AH tại O. Chứng minh rằng \dfrac{{{S_{AOM}}}}{{{S_{ADC}}}} = {\sin ^2}B.{\sin ^2}C

Câu 6: Tìm giá trị lớn nhất của biểu thức: P = \sqrt {x - 7} + \sqrt {14 - x}

B. Đáp án Đề thi giữa kì 1 lớp 9 môn Toán

Câu 1: Thực hiện phép tính

\begin{array}{l} a)\,2\sqrt 5 - \sqrt {125} - \sqrt {80} + \sqrt {605} \\ b)\,2\sqrt {27} - 6\sqrt {\dfrac{4}{3}} + \dfrac{3}{5}\sqrt {75} \\ c)\,\,\dfrac{2}{{\sqrt 6 - 2}} + \dfrac{2}{{\sqrt 6 + 2}} + \dfrac{5}{{\sqrt 6 }} \end{array}

Hướng dẫn giải

\begin{array}{l}a)\,2\sqrt 5 - \sqrt {125} - \sqrt {80} + \sqrt {605} \\ = 2\sqrt 5 - 5\sqrt 5 - 4\sqrt 5 + 11\sqrt 5 \\ = 4\sqrt 5 \\b)\,2\sqrt {27} - 6\sqrt {\dfrac{4}{3}} + \dfrac{3}{5}\sqrt {75} \\ = 6\sqrt 3 - 4\sqrt 3 + 3\sqrt 3 = 5\sqrt 3 \\c)\,\,\dfrac{2}{{\sqrt 6 - 2}} + \dfrac{2}{{\sqrt 6 + 2}} + \dfrac{5}{{\sqrt 6 }}\\ = \dfrac{{2\left( {\sqrt 6 + 2} \right)}}{{\left( {\sqrt 6 - 2} \right)\left( {\left( {\sqrt 6 + 2} \right)} \right)}} + \dfrac{{2\left( {\sqrt 6 - 2} \right)}}{{\left( {\sqrt 6 + 2} \right)\left( {\sqrt 6 - 2} \right)}} + \dfrac{{5\sqrt 6 }}{{\sqrt 6 .\sqrt 6 }}\\ = \dfrac{{2\left( {\sqrt 6 + 2} \right)}}{4} + \dfrac{{2\left( {\sqrt 6 - 2} \right)}}{4} + \dfrac{{5\sqrt 6 }}{6}\\ = \dfrac{{\left( {\sqrt 6 + 2} \right)}}{2} + \dfrac{{\left( {\sqrt 6 - 2} \right)}}{2} + \dfrac{{5\sqrt 6 }}{6}\\ = 2\sqrt 6 + \dfrac{{5\sqrt 6 }}{6} = \dfrac{{5\sqrt 6 + 12\sqrt 6 }}{6} = \dfrac{{17\sqrt 6 }}{6}\end{array}

Câu 2: Giải phương trình:

\begin{array}{l} a)\,2\sqrt {2x + 1} - \dfrac{3}{4}\sqrt {8x + 4} = \sqrt {\dfrac{{18x + 9}}{4}} - 3\\ b)\,\,x - \sqrt {2x + 3} = 0\\ c)\,\sqrt {{x^2} + 4} = \sqrt {3x + 8} \end{array}

Hướng dẫn giải

\begin{array}{l} a)\,2\sqrt {2x + 1} - \dfrac{3}{4}\sqrt {8x + 4} = \sqrt {\dfrac{{18x + 9}}{4}} - 3\\ \Leftrightarrow 2\sqrt {2x + 1} - \dfrac{3}{4}\sqrt {8x + 4} - \sqrt {\dfrac{{18x + 9}}{4}} = 3\\ \Leftrightarrow 2\sqrt {2x + 1} - \dfrac{3}{4}\sqrt {4\left( {2x + 1} \right)} - \sqrt {\dfrac{{9\left( {2x + 1} \right)}}{4}} = 3\\ \Leftrightarrow 2\sqrt {2x + 1} - \dfrac{3}{2}\sqrt {\left( {2x + 1} \right)} - \dfrac{3}{2}\sqrt {2x + 1} = 3\\ \Leftrightarrow - \sqrt {2x + 1} = 3\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\left( {DK:2x + 1 \ge 0 \Leftrightarrow x \ge \dfrac{1}{2}} \right)\\ \Leftrightarrow {\left( { - \sqrt {2x + 1} } \right)^2} = {3^2}\\ \Leftrightarrow 2x + 1 = 9\\ \Leftrightarrow x = 4 \end{array}

Vậy phương trình có nghiêm x = 4

\begin{array}{l} b)\,\,x - \sqrt {2x + 3} = 0\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\left( {DK:\left\{ \begin{array}{l} 2x + 3 \ge 0\\ x \ge 0 \end{array} \right. \Leftrightarrow x \ge 0} \right)\\ \, \Leftrightarrow x = \sqrt {2x + 3} \\ \Leftrightarrow {x^2} - 2x - 3 = 0\\ \Leftrightarrow {x^2} - 3x + x - 3 = 0\\ \Leftrightarrow x\left( {x - 3} \right) + \left( {x - 3} \right) = 0\\ \Leftrightarrow \left( {x + 1} \right)\left( {x - 3} \right) = 0\\ \Leftrightarrow \left[ \begin{array}{l} x + 1 = 0\\ x - 3 = 0 \end{array} \right. \Leftrightarrow \left[ \begin{array}{l} x = - 1\,\,\,\,\left( l \right)\\ x = 3 \end{array} \right. \end{array}

Vậy phương trình có nghiệm x = 3

\begin{array}{l}c)\,\sqrt {{x^2} + 4} = \sqrt {3x + 8} \,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\left( {DK:3x + 8 \ge 0 \Leftrightarrow x \ge \dfrac{{ - 8}}{3}} \right)\\ \Leftrightarrow {x^2} + 4 = 3x + 8\\ \Leftrightarrow {x^2} - 3x - 4 = 0\\ \Leftrightarrow x\left( {x + 1} \right) - 4\left( {x + 1} \right) = 0\\ \Leftrightarrow \left( {x - 4} \right)\left( {x + 1} \right) = 0\\ \Leftrightarrow \left[ \begin{array}{l}x = 4\\x = - 1\end{array} \right.\,\,\,\left( {t/m)} \right)\end{array}

Vậy phương trình có nghiệm x = -1 ; x= 4

Câu 3: Cho hai biểu thức A = \dfrac{{\sqrt x + 7}}{{\sqrt x - 1}}  và B = \dfrac{1}{{\sqrt x + 2}} + \dfrac{3}{{1 - \sqrt x }} + \dfrac{{x + 8}}{{x + \sqrt x - 2}} ( với )

a) Tính giá trị của A biết x = 16

b) Rút gọn B

c) Tìm các giá trị nguyên của x để P = A. B có giá trị nguyên

Hướng dẫn giải

a) Với x = 16 thì A = \dfrac{{\sqrt x + 7}}{{\sqrt x - 1}} \Rightarrow A = \dfrac{{\sqrt {16} + 7}}{{\sqrt {16} - 1}} = \dfrac{{11}}{3}

b)

\begin{array}{l} B = \dfrac{1}{{\sqrt x + 2}} + \dfrac{3}{{1 - \sqrt x }} + \dfrac{{x + 8}}{{x + \sqrt x - 2}}\\ B = \dfrac{1}{{\sqrt x + 2}} - \dfrac{3}{{\sqrt x - 1}} + \dfrac{{x + 8}}{{\left( {\sqrt x + 2} \right)\left( {\sqrt x - 1} \right)}}\\ B = \dfrac{{\sqrt x - 1}}{{\sqrt x + 2}} - \dfrac{{3\left( {\sqrt x + 2} \right)}}{{\sqrt x - 1}} + \dfrac{{x + 8}}{{\left( {\sqrt x + 2} \right)\left( {\sqrt x - 1} \right)}}\\ B = \dfrac{{\sqrt x - 1 - 3\sqrt x - 6 + x + 8}}{{\left( {\sqrt x + 2} \right)\left( {\sqrt x - 1} \right)}}\\ B = \dfrac{{x - 2\sqrt x + 1}}{{\left( {\sqrt x + 2} \right)\left( {\sqrt x - 1} \right)}} = \dfrac{{{{\left( {\sqrt x - 1} \right)}^2}}}{{\left( {\sqrt x + 2} \right)\left( {\sqrt x - 1} \right)}} = \dfrac{{\left( {\sqrt x - 1} \right)}}{{\left( {\sqrt x + 2} \right)}} \end{array}

c) Ta có: P = A.B = \dfrac{{\sqrt x + 7}}{{\sqrt x - 1}}.\dfrac{{\left( {\sqrt x - 1} \right)}}{{\left( {\sqrt x + 2} \right)}} = \dfrac{{\sqrt x + 7}}{{\sqrt x + 2}} = \dfrac{{\sqrt x + 2 + 5}}{{\sqrt x + 2}} = 1 + \dfrac{5}{{\sqrt x + 2}}

Để P nguyên thì \sqrt x + 2 \in {Ư_5} = \left\{ { \pm 1; \pm 5} \right\}

Với

\begin{array}{l} \sqrt x + 2 = 1 \Rightarrow \sqrt x = - 1\left( l \right)\\ \sqrt x + 2 = 1 \Rightarrow \sqrt x = - 3\left( l \right)\\ \sqrt x + 2 = 5 \Rightarrow \sqrt x = 3 \Rightarrow x = 9\\ \sqrt x + 2 = - 5 \Rightarrow \sqrt x = - 7\left( l \right) \end{array}

Vậy x = 9 thì P = A. B có giá trị nguyên

Câu 4: Một chiếc máy bay cất cánh. Đường bay lên tạo với phương ngang một góc sau khi bay được quãng đường 9 km thì máy bay đã bay lên được độ cao là bao nhiêu km theo phương thẳng đứng

Hướng dẫn giải

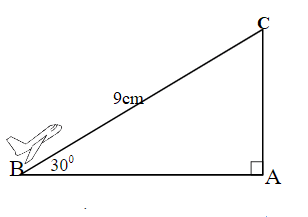
Xét tam giác vuông tại A ta có:

\sin \widehat B = \dfrac{{AC}}{{BC}} \Rightarrow \sin {30^ \circ } = \dfrac{{AC}}{9} \Rightarrow AC = \sin {30^ \circ }.9 = 4,5\left( {km} \right)

Vậy máy bay đã bay được 4,5 km so với phương thẳng đứng

Câu 5: Cho tam giác ABC nhọn có đường cao AH. Gọi M là hình chiếu của H lên AB

a) Biết AM = 3 cm; BM = 9 cm. Tính AH; HM

b) Kẻ IM vuông góc với AC tại N. Chứng minh AB. AM = AC. AN

c) Đường thẳng qua A vuông góc với MN cắt BC tại D; MN cắt AH tại O. Chứng minh rằng \dfrac{{{S_{AOM}}}}{{{S_{ADC}}}} = {\sin ^2}B.{\sin ^2}C

Hướng dẫn giải

a) Ta có: AB = AM + BM = 3 + 9 = 12 cm

Áp dụng hệ thức lượng trong tam giác ABH vuông tại H ta được:

\begin{array}{l} + \,\,A{H^2} = AM.AB\\ A{H^2} = 3.12\\ A{H^2} = 36\\ AH = 6\\ + \,\,H{M^2} = AM.BM\\ H{M^2} = 3.9\\ H{M^2} = 27 \Rightarrow HM = \sqrt {27} \end{array}

b) Áp dụng hệ thức lượng trong tam giác ABH có: AM.AB = A{H^2}(1)

Áp dụng hệ thức lượng trong tam giác AHC có: AN.AC = A{H^2}(2)

Từ (1) và (2) ta được AM.AB = AN.AC = A{H^2}

c) Gọi K là giao điểm của AD và MN

Ta có: \dfrac{{AM}}{{AC}} = \dfrac{{AN}}{{AB}}

Dễ dàng chứng minh được \Delta AMN \sim \Delta ACB\, có:

\widehat {BAC}là góc chung

\dfrac{{AM}}{{AC}} = \dfrac{{AN}}{{AB}}

\Rightarrow \Delta AMN \sim \Delta ACB\,\left( {c - g - c} \right) \Rightarrow \widehat {ANK} = \widehat {ABI};\widehat {ACD} = \widehat {AMO}\,\,\,(1)

Mà \widehat {CAD} = \widehat {ANK}\, = \,\,{90^ \circ }

\widehat {MAO} + \widehat {ABI} = {90^ \circ } \Rightarrow \widehat {MAO} = \widehat {CAD\,\,\,}\left( 2 \right)

Từ (1) và (2) ta được

\begin{array}{l} \Delta ADC \sim \Delta AOM\left( {g - g} \right)\\ \Rightarrow \dfrac{{{S_{AOM}}}}{{{S_{ADC}}}} = {\left( {\dfrac{{AM}}{{AC}}} \right)^2} = {\left( {\dfrac{{AM}}{{AI}}.\dfrac{{AI}}{{AC}}} \right)^2} = {\left( {\dfrac{{AM}}{{AI}}} \right)^2}.{\left( {\dfrac{{AI}}{{AC}}} \right)^2}\\ = co{s^2}\widehat {NAO}.{\sin ^2}C = {\sin ^2}{\bf{B}}{\sin ^2}C \end{array}

Câu 6: Tìm giá trị lớn nhất của biểu thức: P = \sqrt {x - 7} + \sqrt {14 - x}

Hướng dẫn giải 

\begin{array}{l} P = \sqrt {x - 7} + \sqrt {14 - x} \\ \Rightarrow {P^2} = {\left( {\sqrt {x - 7} + \sqrt {14 - x} } \right)^2}\\ {P^2} = \,\,x - 7 + \,14 - x + 2\sqrt {x - 7} .\sqrt {14 - x} \\ {P^2} = \,\,x - 7 + \,14 - x + 2\sqrt {\left( {x - 7} \right)\left( {14 - x} \right)} \\ {P^2} = 7 + 2\sqrt {\left( {x - 7} \right)\left( {14 - x} \right)} \le 7 + x - 7 + 14 - x = 14 \end{array}

{P^2} \le 14 hay  P \le \sqrt {14}

Dấu “ =” xảy ra khi \begin{array}{l} \left( {x - 7} \right) = \left( {14 - x} \right)\\ \Leftrightarrow 2x = 21\\ \Leftrightarrow x = \dfrac{{21}}{2} \end{array}

Vậy GTLN của P = \sqrt {14} khi x = \dfrac{{21}}{2}

Tài liệu liên quan:

  • Đề thi giữa kì 1 Toán 9 - Đề số 4
  • Đề thi giữa kì 1 Toán 9 - Đề số 2


Trên đây Giaitoan.com giới thiệu tới quý thầy cô và bạn đọc tài liệu Đề kiểm tra giữa học kì 1 môn Toán 9 - Đề 3. Ngoài ra học sinh có thể tham khảo thêm một số tài liệu liên quan: Lý thuyết Toán 9, Luyện tập Toán 9, Giải Toán 9 Tập 1, ....

  • 277 lượt xem
Chia sẻ bởi: Lê Thị Thùy
Tìm thêm: Toán 9 Đề thi học kì lớp 9
Sắp xếp theo
Xóa Đăng nhập để Gửi

    Chủ đề liên quan